Random Walks
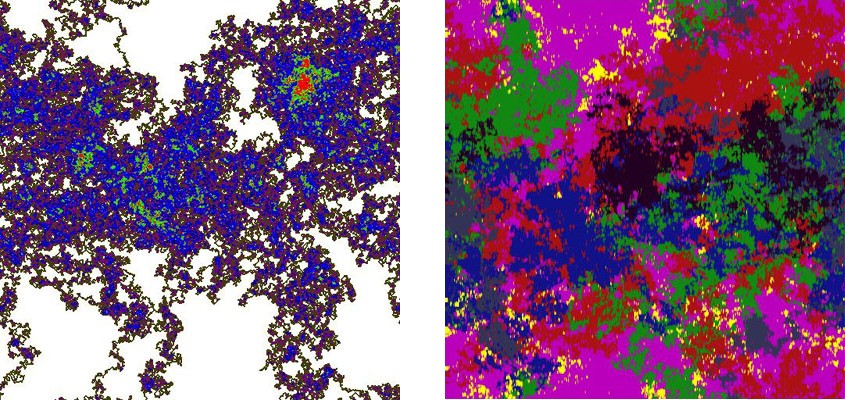
Two simple random walks in the torus. Points are colored according to occupation measure (left) and hitting time (right). Pictures created by Raissa D’Souza.
Description:
Denote by T(x,r) the occupation measure of a disc of radius r around x by planar Brownian motion run till time 1, and let T(r) be the maximum of T(x,r) over x in the plane. We prove in [1] that T(r) is a.s. asymptotic to 2 r2 |log r|2 as r tends to 0, thus solving a problem posed by Perkins and Taylor (1987). Furthermore, for any a<2, the Hausdorff dimension of the set of points x for which T(x,r) is asymptotic to a r2|log r|2, is almost surely 2-a. As a consequence, we prove a conjecture about planar simple random walk due to Erdős and Taylor (1960): The number of visits to the most frequently visited lattice site in the first n steps of the walk, is asymptotic to (log n)2/pi. We also show that for a between 0 and 1/pi, the number of points visited more than a(log n)2 times in the first n steps, is approximately n{1-a pi}.
Hitting time results:
References:
-
Thick points for planar Brownian motion and the Erdos-Taylor conjecture on random walk. (A. Dembo, Y. Peres, J. Rosen and O. Zeitouni). Acta Math. 186 no. 2, (2001), 239–270.
-
Cover Times for Brownian Motion and Random Walks in Two Dimensions. (A. Dembo, Y. Peres, J. Rosen, and O. Zeitouni). Ann. Math., 160 (2004).
-
Late Points for Random Walks in Two Dimensions. (A. Dembo, Y. Peres, J. Rosen, O. Zeitouni). Annals of Probability. To appear.